Mixed / chevron baffles fishway

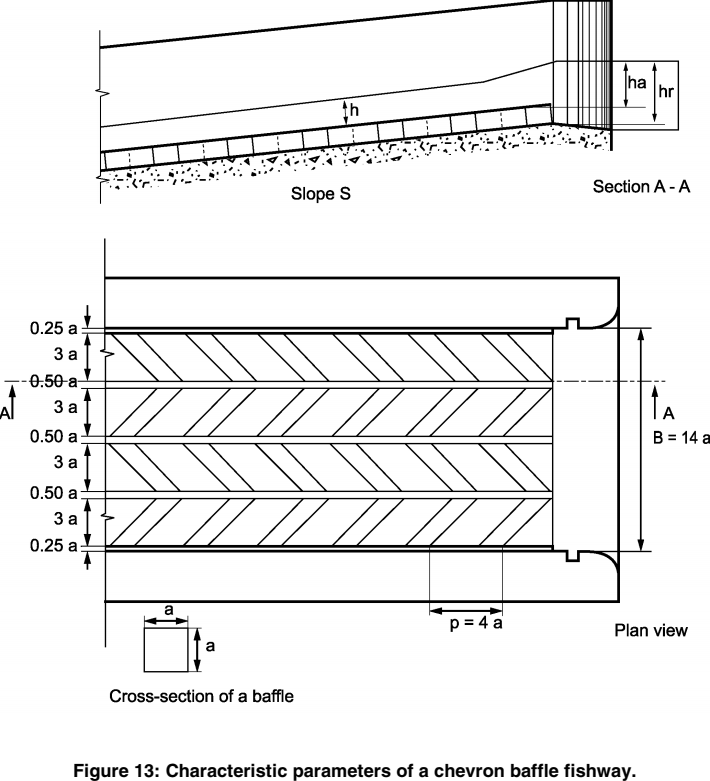
Excerpt from Larinier, 2002¹

Hydraulic laws given by abacuses

Experiments conducted by Larinier, 2002¹ allowed to establish abacuses that link adimensional flow \(q^*\) :

\[ q^* = \dfrac{Q/L}{\sqrt{2g}a^{1,5}} \]

to upstream head \(ha\) and the average water level in the pass \(h\) :

Abacuses of a mixed / chevron baffles fishway for a slope of 10% (Excerpt from Larinier, 2002¹)

Abacuses of a mixed / chevron baffles fishway for a slope of 15% (Excerpt from Larinier, 2002¹)

To run calculations for all slopes between 8% and 22%, polynomes coefficients of abacuses above are themelves adjusted in the form of slope \(S\) depending polynomes.

We thus have:

\[ ha/a = a_2(S) q^{*2} + a_1(S) q^* + a_0(S) \]

\[a_2(S) = 0.188324S - 0.0427461\]

\[a_1(S) = - 2.47998S + 1.25363\]

\[a_0(S) = 5.02138S + 0.709434\]

And:

\[ h/a = b_2(S) q^{*2} + b_1(S) q^* + b_0 \]

\[b_2(S) = - 0.0733832S - 0.00839864\]

\[b_1(S) = 0.176261S + 0.661656\]

\[b_0(S) = - 4.97686S + 1.30546\]

Calculation of \(ha\), \(h\) and \(Q\)

We can then use those coefficients to calculate \(ha\), \(h\) and \(q^*\):

\[ ha = a \left( a_2 (q^*)^2 + a_1 q^* + a_0 \right)\]

\[ h = a \left( b_2 (q^*)^2 + b_1 q^* + b_0 \right)\]

Using the positive inverse function, depending on \(ha/L\), we get:

\[ q^* = \dfrac{-a_1 + \sqrt{a_1^2 - 4 a_2 (a_0 - h_a/a)}}{2 a_2}\]

And we finally have:

\[ Q = L q^* \sqrt{g} a^{1,5} \]

Calculation limitations of \(q^*\), \(ha/a\) and \(h/a\) are determined based on the extremities of the abacuses curves.

Flow velocity

Flow velocity \(V\) corresponds to the minimum flow speed given the flow section \(A_w\) at the perpendicular of the baffle :

\[ V = \dfrac{Q}{A_w} \]

for mixed / chevron baffles fishways using the notation of the schema above, we have:

\[ A_w = h \times L\]

Upstream apron elevation \(Z_{r1}\)

\[ Z_{r1} = Z_{d1} + \frac{3 a S - a}{\sqrt{1 + S^2}} \]

---

1. Larinier, M. 2002. “BAFFLE FISHWAYS.” Bulletin Français de La Pêche et de La Pisciculture, no. 364: 83–101. doi:10.1051/kmae/2002109. ↩↩↩↩